LAUSR

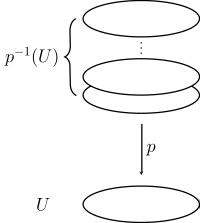
This paper contributes to the classification of finite 2-arc-transitive graphs. In [12], all the regular covers of complete bipartite graphs Kn,n were classified, whose covering transformation group is cyclic and whose fibre-preserving automorphism group acts 2-arc-transitively. In this paper, a further classification is achieved for all the regular covers of Kn,n, whose covering transformation group is elementary abelian group of order p2 and whose fibre-preserving automorphism group acts 2-arc-transitively. As a result, two new infinite families of 2-arc-transitive graphs are found. Moveover, it will be explained that it seems to be infeasible to classify all such covers when the covering transformation group is an elementary abelian group of order pk for an arbitrary integer k.