LAUSR

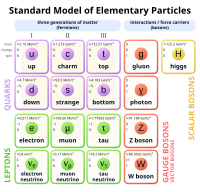
The observed hierarchical charged fermion masses of three generations seem to imply that these masses are originally universal for three generations and then get exponential suppression with "quantized exponents" by some mechanism. We argue that such remarkable feature of hierarchical fermion mass spectrum may be naturally understood in the scenario of gauge-Higgs unification, where the universality of fermion masses is guaranteed by the fact that Higgs boson is originally gauge boson in this scenario and also the quantized exponents may be attributed to the well-known quantization condition of magnetic charge of the magnetic monopole placed inside the torus, as the extra dimensional space. Because of the presence of the magnetic monopole, we get a chiral theory and multiple Kaluza-Klein zero modes even if we introduce only a single 6-dimensional Weyl fermion. We present two types of three generation model, which succeed to realize the remarkable hierarchical mass spectrum.