LAUSR

Sparsest representations and approximations of an underdetermined linear system

Sign Up to like & get
recommendations!
0 Published in 2018 at "Inverse Problems"

DOI: 10.1088/1361-6420/aab246

Abstract: In an underdetermined linear system of equations, constrained l1 minimization methods such as the basis pursuit or the lasso are often used to recover one of the sparsest representations or approximations of the system. The… read more here.

Keywords: linear system; sparsest representations; basis pursuit; underdetermined linear ... See more keywords

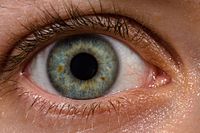
Basis Pursuit With Sparsity Averaging for Compressive Sampling of Iris Images

Sign Up to like & get
recommendations!
3 Published in 2022 at "IEEE Access"

DOI: 10.1109/access.2022.3140429

Abstract: This paper proposes novel compressive sampling (CS) of colored iris images using three RGB iterations of basis pursuit (BP) with sparsity averaging (SA), called RGB-BPSA. In RGB-BPSA, a sparsity basis is performed using an average… read more here.

Keywords: basis pursuit; basis; iris images; pursuit sparsity ... See more keywords

Basis Pursuit Anisotropic Inversion Based on the L1–L2-Norm Regularization

Sign Up to like & get
recommendations!
1 Published in 2022 at "IEEE Geoscience and Remote Sensing Letters"

DOI: 10.1109/lgrs.2021.3075062

Abstract: Prestack seismic inversion for VTI media (transversely isotropic with vertical axis of symmetry) is a technique that can be useful to obtain the properties (velocity, density, and anisotropy parameters) of shale reservoirs. Since conventional inversion… read more here.

Keywords: basis pursuit; inline formula; inversion; tex math ... See more keywords

Basis Pursuit Denoising via Recurrent Neural Network Applied to Super-Resolving SAR Tomography

Sign Up to like & get
recommendations!
2 Published in 2023 at "IEEE Transactions on Geoscience and Remote Sensing"

DOI: 10.1109/tgrs.2022.3221185

Abstract: Finding sparse solutions of underdetermined linear systems commonly requires the solving of $L_{1}$ regularized least-squares minimization problem, which is also known as the basis pursuit denoising (BPDN). They are computationally expensive since they cannot be… read more here.

Keywords: neural network; basis pursuit; recurrent neural; pursuit denoising ... See more keywords

Compressed Sensing Using Binary Matrices of Nearly Optimal Dimensions

Sign Up to like & get
recommendations!
0 Published in 2020 at "IEEE Transactions on Signal Processing"

DOI: 10.1109/tsp.2020.2990154

Abstract: In this paper, we study the problem of compressed sensing using binary measurement matrices and $\ell _1$-norm minimization (basis pursuit) as the recovery algorithm. We derive new upper and lower bounds on the number of… read more here.

Keywords: binary matrices; sensing using; basis pursuit; using binary ... See more keywords